|
This week I visited a Grades 3 and Grades 3/4 class at Betty Huff Elementary. The two teachers and I met during the first week of school and worked collaboratively to discuss how to begin the year. I am working with these teachers this term to support their professional inquires which focus on developing their students curricular competencies in relation to content, as well exploring what inquiry-based practices could look like in mathematics. We began by creating a mini-unit exploring the following questions: What is math? What does it mean to do math? Where does math live in the world? Additionally, we wanted students to begin to explore their own identity as a math learner. This is an important aspect of BC's redesigned curriculum. The Positive Personal and Cultural Identity is one of our core competencies and includes the awareness, understanding, and appreciation of all the facets that contribute to a healthy sense of oneself . We wondered how our students saw themselves as Mathematicians.  We began with an activity I had seen on Graeme Anshaw's blog where he asked students to share their personal histories with mathematics. The teachers gave each student a sticky and ask them to write or draw how they felt about math and why. Then students were invited to share their thoughts with the class and place their sticky on the line spectrum. The teachers commented how through this activity they were able to learn about the mindsets students held with regard to mathematics. As you can see the Grade 3/4 class held more positive views, while the Grade 3 class was more mixed. The teachers remarked how curious they were to dig more deeply into these stories and work with these students to shift some of their negative feelings. I visited the class on the second day of the mini-unit. We began with the question "What is Mathematics?", and surprisingly, we learned that not all children had an understanding of the word "Mathematics". They didn't realize it was just a longer version of the word "Math"... an important reminder that all children are "mathematics language learners" and we must pay attention to the language we use. When reflecting upon the charts, we were able to see similarities to the work that Tracy Zager and Deborah Nichols reported hearing from a Grades 1/2 class (pg. 11 - 13 in Becoming The Math Teacher You Wish You'd Had). It seemed that our students felt mathematics was mostly about computation. Their experiences had been many worksheets, which they felt was really hard. Inspired by Tracy Zager's suggestions to use books to help expand children's view of mathematics, we read aloud On A Beam of Light: A Story of Albert Einstein by Jennifer Berne. Before beginning the book, we passed out "Notice/Wonder" sticks to the students. We asked them to raise their "Notice" glasses if they noticed anything in the book that they thought represented mathematics, which we would add to the chart. Additionally, student were asked to raise their "Wonder" question marks if they thought they might have seen or heard something that could be added to our chart, but were unsure. Credits go out to Beth Kobett who shared the idea of Notice and Wonder sticks on Twitter.
Below is the mini-unit we created including using ideas from Tracy Zager's book, Jo Boaler's inspirational week of maths, and Graeme Anshaw's blog. Feel free to leave any feedback you have on our mini-unit. We welcome your ideas and suggestions! I am curious how did you start your year with your Mathematicians?
0 Comments
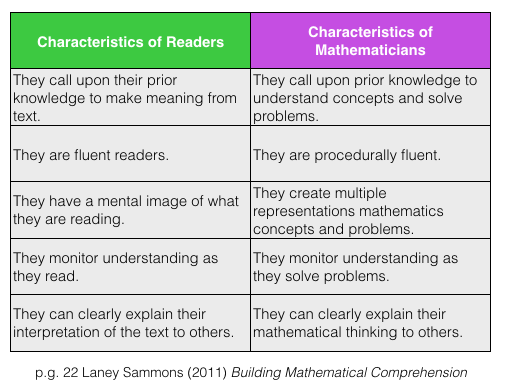
Friday was the Provincial Professional Development day in our province, BC. I had the pleasure of co-presenting with Lisa Schwartz, Richmond School District's Literacy Consultant. You might be asking yourself why a math educator like myself would be presenting with someone with a literacy background. Well, not only are we friends, but over the years in our discussions about teaching, we have come to realize that their are many commonalities between developing readers and mathematicians. In our presentation we shared many of our favourite children's books and how we have used them for both literacy and mathematical learning intentions. In Laney Sammons book Building Mathematical Comprehension: Using LIteracy Strategies to Make Meaning, she uses a chart to discuss the similarities readers and mathematians share. These similarities are yet another reminder that our habits of mind, or core and curricular competencies, as now called in our revised BC Curriculum, are important! In order to develop as mathematicians or readers, our students need to:
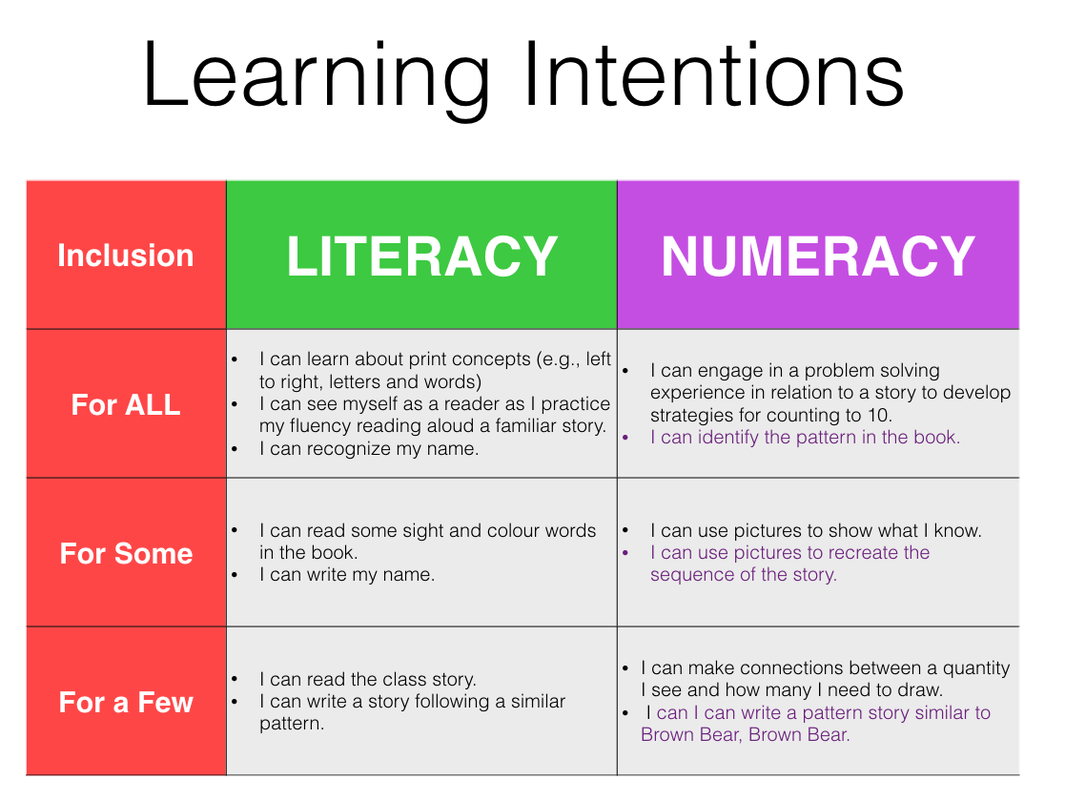
It is this knowledge about the importance of our processes to develop understanding, that is one of the central tenets at the heart of the new curriculum. Our curriculum is no longer solely focused on content. Although, I would argue it never was. The processes in the 2007 BC Math IRP mirror many of the curricular competencies, but through the physical design of the "Know, Understand, Do" (KUD) model, the curricular compentencies have been lifted and made a priority. Using this framework, as well as a belief that learning needs to include all of our children, Lisa and I worked with several children's literature books to create learning intentions for both literacy and numeracy. The following is an example of one of the books I used. Brown Bear, Brown Bear, What Do You See? by Bill Martin Jr. is a classic picture book that students and teachers have enjoyed reading aloud for years. Using the book, we designed learning intentions for all, some and a few, as you can see below. Working with a Kindergarten class, I did an interactive read aloud and engaged with the book prior, during, and after reading to make predictions, ask questions, explore mathematical ideas, and engage in problem solving. Hintz and Smith (2013) describe this process as "mathematizing". Mathematizing is a way of looking at the world through a mathematical lens. Through reading motivating children's literature books, students are inspired to read and write. They are also able to make connections and build their schema in meaningful ways.  The Kindergarten teacher and I were not sure if the students would be able to represent how many animals they saw in the story. When we used the concrete felt animals the students could touch and count, using one-to-one correspondence to count and tell how many (cardinality). Once back at their tables, it was going to be more complex to represent what they were seeing and hearing in the book. We were excited to see the different strategies the students might think of to use. Some used tally marks but without a diagonal line crossing over four vertical lines. A couple students drew pictures, and a few used checkmarks. Their representations provided excellent assessment for learning because we could see the child's sense-making through the images they created. We made note of a few children who were beginning to use numerals to tell information. As well, by reflecting on the emerging tally marks, we knew one of our next steps would be to explicitly teach how we organize tally marks. The representations also provided us a record that we can use for assessment, to compare to later in the year to note any differences and growth. Following this lesson, the students enjoyed reading the story once again and following it up with sequencing the animals in the story. Everyone had fun reading the story aloud as a class, while touching their sequencing strips. This familiar story gave all of the students an opportunity to see themselves as readers. Later in the week, the Kindergarten teacher worked collaboratively with the students to co-create a class book, with a similar pattern. This new, class authored book is now a class favourite. What I was reminded of while planning for this presentation, is that teachers do not have to simply use books with explicit mathematical concepts to explore math concepts. Instead, I believe almost any book could be used because when you dig deep enough, in my experience, math can be found in just about any situation. What favourite books have you used with your young Mathematicians? I am always looking for new ideas. Hintz, A. and Smith, A. Mathematizing Read Alouds in Three Easy Steps. In Reading Teacher. 67(2), May 2013. Sammons, L. (2011). Building mathematical comprehension:. Huntington Beach, CA: Shell Education.  I love returning to school in September. I get giddy with excitement purchasing school supplies and can't wait to meet my new students. This year my two kids have started Grades 6 and 8. They also share my love of picking out colourful pencils, pens, binders, and such, but since we didn't know what was required for high school I told my son we would purchase his supplies at the end of the first week. Last weekend, I took the necessary time to review my son's course outlines. Generally they were as I expected but it was a comment from my son that caused me pause. He told me that he needed more pencils and a bigger eraser for Math. He said that all his Math work had to be done in pencil and the erasers at the end of pencil weren't going to be big enough to erase mistakes. Herein, is where my mind started buzzing with questions. This long-standing tradition of using only pencil in Mathematics so students can erase their errors doesn't make any sense to me! We don't insist on using only pencil in any other subjects, so why Math? In writing, when students review and revise their work, they simply write on top or under what they have written, cross one word out and add another and add indentation marks to show where they have added. This makes it possible for teachers to see the trajectory of learning. Likewise, when children read to us and they make a mistake, we don't halt them and say "stop, that's incorrect"; instead we give them time to see if they catch their mistake and self-correct and then we discuss the error and strategies to help the student. The practice of writing in pencil so that we can erase mistakes in Math runs contradictory to all the current research we know about developing growth mindsets, honouring process over product, and capitalizing on opportunities to move our students' learning forward. Jo Boaler writes Studies of successful and unsuccessful business people show something surprising: what separates the more successful people from the less successful people is not the number or successes but the number of mistakes they make, with the more successful people making more mistakes (Mathematical Mindsets, 2016, p.g. 15) If mistakes are a necessary part of our learning process, then why are Math teachers asking children to erase them? I believe mistakes should be seen positively, as they show what the child currently understands and any misconceptions are opportunities for learning to occur. Furthermore, we know from both research and experience that when we build classroom communities that are safe to take risks and use errors for instruction, we have the potential to increase our students' understanding (Bray, 2013). Why would we give up this opportunity by encouraging erasing? I have wrestled with this idea before. Two years ago I sat frustrated trying to figure out my son's understanding about adding fractions with different denominators.I knew he had some misconceptions but I couldn't make sense of his thought process because the work he brought home from school had been fully erased. He had shown his teacher his work and was told to erase it and re-do it. I then asked his teacher to please refrain from having my son erase and explained my rationale. Here we are two years later with more knowledge about the importance of mistakes as part of learning and I am unsure if we are further ahead. I would like to be able to tell you that both my children are using only pen in their Math classes but that wouldn't be the truth. They don't want to stand out against their peers or make their teachers unhappy so they continue to use pencil... but they no longer erase (at least that I can see). As I move forward supporting both teachers and students in Math classes this year, I will be bringing with me a beautiful tin of colourful pens, with all sorts of interesting designs, including some that are smelly! We will use these to record our Mathematical thinking and understanding and there will be NO ERASING! All thoughts will be valued and mistakes will be seen positively, as part of our learning and growing process! I am interested to hear others thoughts on this topic and the role pencils and erasers play in your Mathematics classes. Please feel free to add a comment. Boaler, J. (2016). Mathematical mindsets: Unleashing students' potential through creative math, inspiring messages, and innovative teaching. Bray, Wendy S. 2013. "How to Leverage the Potential of Mathematical Errors." Teaching Children Mathematics 19 (7): 424-431.  A couple of days ago I tweeted that I thought I was going to like this resource and some folks asked me to let them know my thoughts. Well, as I thought, this book did not disappoint! Authors Linda Dacey, Karen Gartland, and Jayne Bamford Lynch share some engaging new number games and puzzles and twists on old favourites like "Go Fish Numbers" (e.g., students search for "packs" of three matching representations of a given number). They also share a few free online games for each conceptual area. Although I really liked the games/puzzles in the book, what impressed me the most were the points they raised around the teaching of games/puzzles; these were highly insightful! Some of my take aways from the book include:
My only suggestion (and this is for the editor) - is that I wish there was an online url link where I could go and print out the blackline masters in the standard 8.5 x 11 size. It is just a minor detail but one that would save me time and as teacher, that is something we could all use more of. I highly recommend this resource and intend to use it this coming year!  It has now been eight months since I began my position as an Early Numeracy Teacher in Surrey. My learning curve has been steep this year and I have loved every minute of it. I am trying new things and constantly reflecting and deepening my understanding of how children develop number sense. This learning brought me a renewed energy and passion for teaching. One of the many activities I have been working with this year, is "Counting Collections". I initially read about it in Math Exchanges by Kassia Omohundro Wedekind and decided to give it a try. Quickly I saw the rich learning that resulted from this activity. Students were able to show their understanding of:
I began by reading aloud the book How Many Snails by Paul Giganti. The children enjoyed counting the different items on each page. After reading a few pages, we discussed how challenging it was to count some of the items, as the items were disorganized on the pages. I asked how the illustrator could have made the items easier to count. The child had lots of great ideas suggesting he could group the items. Next I shared with the students that we would be using different math "tools" to assist us in counting items in an organized way. I modelling with the class as a whole group. I took out a bag of items and placed them on the carpet. The students provided several estimates of how many they thought there were and then I showed the students a few math "tools" that I thought they might find helpful in assisting us to count the items. I did not tell the students how to use the tools. Instead I showed them an ice-cube tray and passed it around and asked if they had any ideas how we could use it to help us. One student suggested putting an item in each spot and then showed how we could count by 2's. I did the same thing with other "tools" such as an image of a hand, which the students noticed could be used to count items in groups of five. I found the best way to organize by collections was to use the $4.99 clear totes from Michaels. I have three totes with baggies of items, ranging in numbers from 1 - 20, 21 - 50, and 51 - 100. I also have lots of different mathematical "tools" for the students to use to organize their items and assist them with counting, such as:
After doing several examples with the class, I modelled how to work with a partner to count a collection. Together both students determine a plan for how they will count their items. Next they organize their items. The students can count together and then independently each of them checks their count. Once they believe they know how many, they record the numeral on a sticky note. Early on in the year, the students needed number lines or hundreds charts to help them know what the corresponding numeral looked like. Although they may have been able to orally say "23" they did not know how to write it. After the students have recorded the numeral corresponding to the set they counted they raise their hand and either myself or the classroom teacher check-in with the students and ask them to explain the strategies they used. As teachers, we often take photos or videos on our iPads to highlight and/or document interesting strategies. We share these with the class during the sharing component and the students take such pride explaining their strategies. Now that we have had many experiences with Counting Collections, the children are given the choice to work alone or in partners. They also have the option of taking items from any of the three totes (1 - 20, 21 - 50, and 51 - 100). Counting Collections allows students to work to their potential or as notable Math educator Robert Kaplinsky likes to say ~ student's need to engage in a productive struggle. Through the sharing of strategies in the last ten minutes of the lesson, students learn different ways to count and inspired to try new tools and group items in different ways. Many Primary teachers are using Counting Collections and have shared their reflections. If you are interested in trying Counting Collections, I recommend reading the following posts as I found them helpful.
 Last weekend I was one of the lucky 1200 people who came together to form a vibrant community of Math educators in Whistler, British Columbia at the Northwest 2015 Conference. All of the sessions that I attended were amazing, but the session that impacted me the most was IGNITE. For those of you not familiar with the term IGNITE, it is a style of presentation in which featured speakers are given five minutes to present on a topic using only 20 slides that auto-advance after 15 seconds. Below is brief synopsis of the the powerful messages I took away from the speakers! MARIAN SMALL - As educators, may we never cease to give a little extra push... CAROLE FULLERTON - Educators need to think and ask themselves "What if... questions?" and be willing to try new things, explore possibilities, and take risks. What if we gave our students opportunities to interact with Mathematics beyond determined grade levels, classroom walls, and text books? ALLISON HINTZ - Our Math community is vibrant. We are constantly learning and listening; Deeply listening is complex. Students listen the way they are heard. Classroom conversations are crucial to learning. Listening well allows us to be responsive educators. JANICE NOVAKOWSKI - Where is the wonder in your Mathematics class? Who's questions are students answering? We don't just play with manipulatives and math tools, we play with ideas. We need to nurture a sense of wonder. ANDREW STADEL - Is your Math class memorable or not? We need more memorable Mathematics? Which graphic best represents your class? ROBERT KAPLINSKY - When learning to ride a bike, we would never tell our kids to get off their bike and let us ride it for them. No, they need to struggle while learning to ride the bike themselves. In Math students need productive struggle. High rewards occur when this happens. How did I live what I learned this week?As mentioned in my bio, I am an Early Numeracy Teacher and have the privilege of working in an inner city school with three wonderful teachers and their amazing students twice each week. In the K/1 and Grade One classes this week, we played with popcorn and ghosts in Math, which was a BIG hit and I hope very memorable (thanks Andrew!). In the K/1 class we had rich discussions about our ghosts (little ghosts in the K/1 class and one big class ghost in the Grade One class). The students shared their wonderings and their questions (thanks Janice!). I listened to their questions and their responses and we were able to explore one of their questions. They wondered how many pieces of popcorn it would take to fill either our little or big ghosts.  When thinking and planning for the Grade One class, I wondered if perhaps the large ghost might be too challenging for the class. Maybe the numbers they would need to count would be too challenging for some? But I remembered that we all need a little push and productive struggle (thanks Marian and Robert!). Before we began, we listened to their estimations. This listening provided a window in the students estimation skills and number sense (thanks Allison!). We provided each learner with a scaffold to assist them in counting their handful of popcorn. The K/1 class used ten frames as they were used to working with these. Their familiarity allowed them to understand when they had ten and some more. We assisted them in counting on beyond ten. I listened with both my eyes and ears as I observed whether students had one-to-one correspondence, and cardinality. The Grade Ones used a hundred's chart that had been blown up onto an 11 x 17 piece of paper. They placed one piece of popcorn on each number and circled the last number they placed a piece of popcorn on. After each student had counted and recorded the number of popcorn they had, they glued their popcorn down on the ghosts (individual ones for the K/1 students and the one class ghost for the Grade Ones).  After the Grade Ones had glued their popcorn on the ghosts we asked them to represent the number of popcorn they had counted and glued down us using base-10 blocks. Then we gathered as a class and discussed how we could determine how many pieces in all were on our class ghost. Although the trading in/regrouping to make tens and hundreds was a new concept for some students and a "productive struggle" (thanks Robert), I saw many students have ah-ha moments. I was grateful we provided a opportunity for a "push" for them (thanks Marian). The above popcorn ghost lessons were inspired and adapted from a lesson in Box it or Bag It Mathematics. My final period of the morning I spend with the Kindergarten class. They had been reading/singing the Five Little Pumpkins poem for the past few days. I wondered how we might bring alive the Math from the poem and create an engaging activity in which the students could all participate instead of just one or two individuals who moved our felt pumpkins on the felt board. Inspired by Kim Suttons' counting rope, I brought in pipe cleaners (thanks Sandra) and some orange beads. The students counted five beads or pumpkins as we called them and placed them on the fence (the pipe cleaner). We reenacted the story several times all together and the students moved their little pumpkins back and forth and told the Math stories (e.g., four and one is five AND when we switch our hands we learn that one and four is also five - the commutative property). The students were so excited with their little pipe cleaners and beads and were able to be creative and tell new and different math stories with their counting line. As I saw the joy in their faces, I wondered "What if I let them take these counting lines home? Would they retell the Five Little Pumpkins poem and share the Math with their families? Would they continue to create Math stories at home?" I decided I needed to see "What if?" and asked each of them to place their "pumpkins on the fence" in their backpacks (Thanks Carole for encouraging me to wonder what if!).  As a parent of two kids, aged 10 and 12 I am learning many things about tweens! I am learning new acronyms for texting, "bad" words I wasn't aware of, and that bathing everyday does not necessarily occur without adult reminders. My new understanding of tweens could fill an entire blog post.. but my children might not appreciate that. This weekend in a discussion with my two tweens, I learned something I wasn't necessary surprised by, but was deeply saddened about. We were talking about the subject of Mathematics and I was asked what they were doing at school. In unison both my children groaned about their dislike for the subject. As a passionate Math teacher, I commented that they just needed to try seeing the beauty of the subject. Both started laughing and said "Mom, don't you know what MATH stands for?" Puzzled I said "no". They then they proceeded to inform me that in their minds, and apparently the minds of "all of their peers" Math stood for "Mental Abuse To Humans". Perhaps I have been living under some rock of Math utopia, but I was surprised that my kid's generation thought this way. I prodded both kids a little further and asked if they really believed this saying about Math? How could my kids not value Math? I mean they have had some experiences at home with me and I know they have had some excellent teachers who have taught Math in engaging ways... But something I have noticed, is that as they have moved up into the intermediate grades much of their experiences with Math have been through interactions with text books. I am not saying that using a text book is all bad or that teachers who use text books are wrong to do so, but I think texts tend to focus on paper and pencil exercises where discrete skills and concepts with little context are the focus. Similarly facts and algorithms are told to students, as opposed to having the learners discover different strategies that work and make sense to them. Texts also do not tend to emphasize collaboration; therefore, much of the work is done in isolation. Looking at Math through these types of experiences, I could see how my kids viewed Math as boring and tortuous (yes, that was a word they used!). Teachers have tough jobs, especially at the Elementary level where they are required to be knowledgeable of many curricular areas. Teachers want to engage students in Mathematics but often either don't have the time to or knowledge of what resources needed to shift their practice. Teacher preparation schools (e.g., Universities) aren't much help either. Often, teacher candidates get less than 24 hours of Math instruction to prepare them to teach Math from Kindergarten to Grade Seven. My point in all this discussion is that teachers like myself, who are lucky enough to focus solely on Math education education, need to create places where we share key resources, ideas, and stories from our classes. Similar to our students, we need to connect, collaborate, and learn from each other. As disappointed as was with my discussion with my kids and their negative disposition to Mathematics, it reminded me of the urgency. We must unite in our plight to develop children who love Mathematics! Hearing my children's voices about Math was just the nudge I needed to push me to finally curate this site... it had been percolating in my mind for awhile. Our Mathematical moments/stories have value and they have the power to inspire others. I am ready to begin sharing my stories. Are you? If you are a Mathematics educator who blogs about your practice, I encourage you to share your stories! We can make positive change! |
About Me
I am a Numeracy Helping Teacher with the Surrey Schools District. Each day I am thankful for being able to work with amazing students and teachers in an area I am passionate about ~ Mathematics! Subscribe:Click the RSS feed button
Archives
October 2018
Categories
All
|
||||||||||||||||||||


















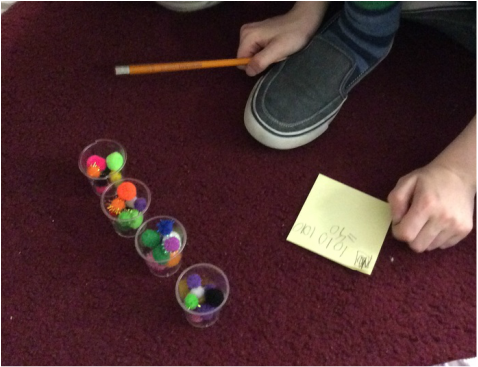







 RSS Feed
RSS Feed
